Maximum Area of a Rectangle With Fixed Perimeter

X2002 100 mm. Arange 0 half step_size.

For Fixed Perimeter Rectangle Square Has Maximum Area Youtube

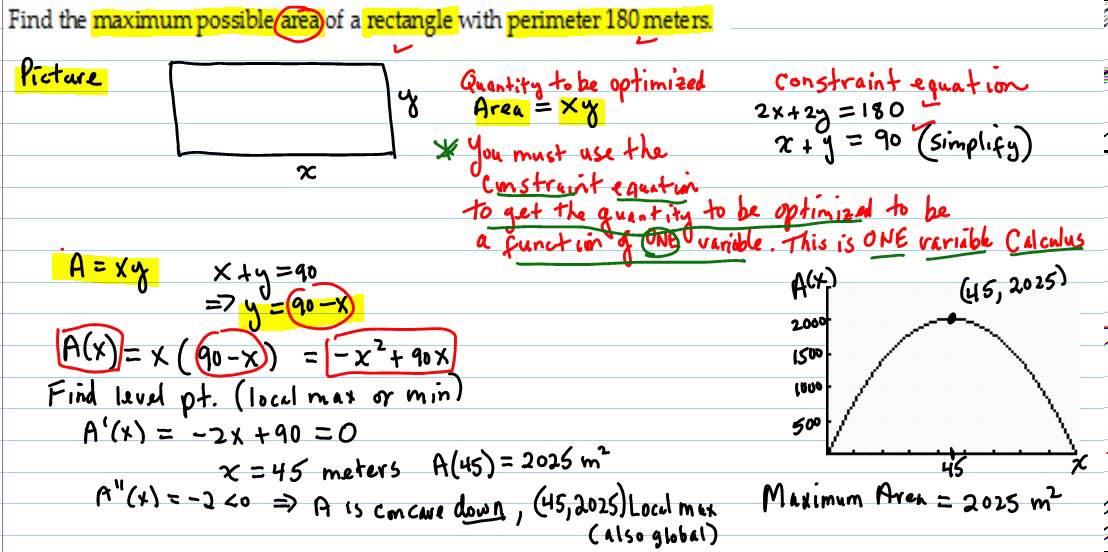
The result you need is that for a rectangle with a given perimeter the square has the largest area.

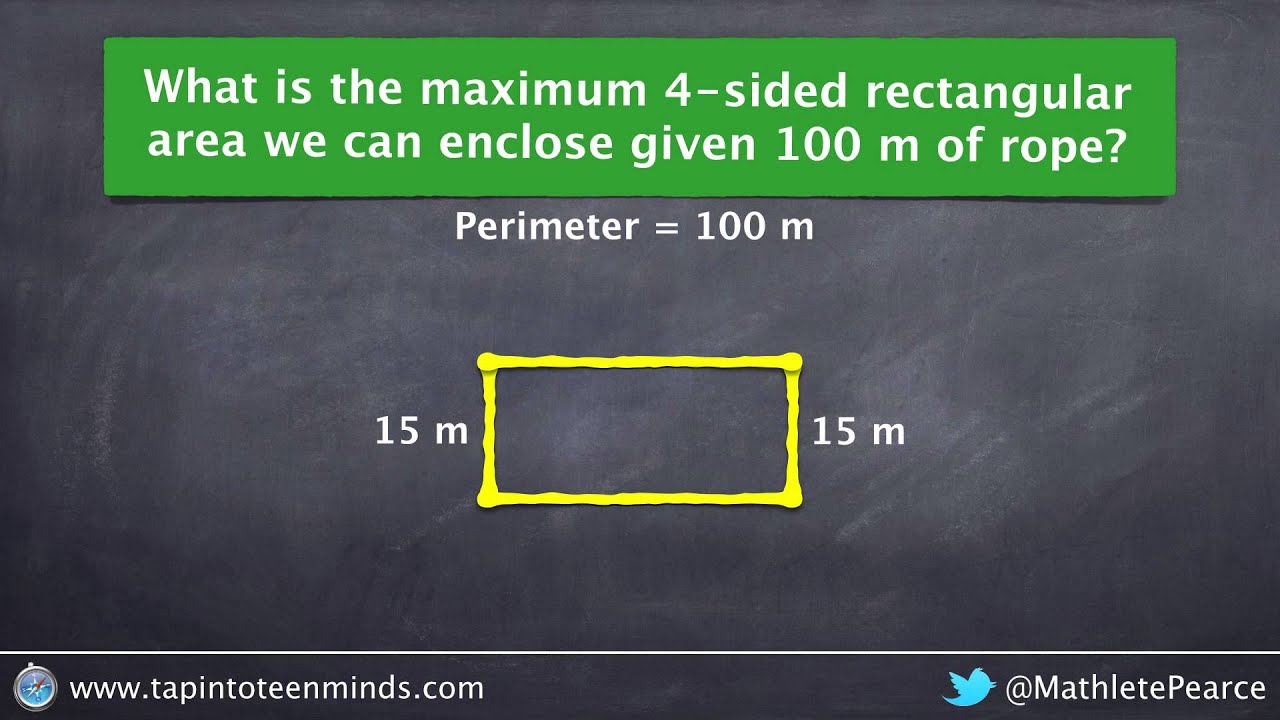
. Predict the dimensions of the rectangle with the largest area. 2 Use slider a to fix the perimeter of 24 units. Answer 1 of 8.

So with a perimeter of 28 feet you can form a square with sides of 7 feet and area of 49 square feet. So the triangle that gives the maximum area for a given perimeter is an equilateral triangle. Then 20 2 l b Thus maximum area of the rectangle that can be formed with fixed perimeter 20 is.

We can do this by setting the derivative to 0 A20-2x0-x10-y10 Just as we thought. 1364 34 so that the side of such a square will be 34 and its area 342 1156. Inside Our Earth Perimeter and Area Winds Storms and Cyclones Struggles for Equality The Triangle and Its Properties.

Axyx20-x20x-x2 And we have to find an extreme for that. 4002 -x 200-x mm. We also know that the area of the rectangle is Alw but we can express this as a function of a single variable.

A -L260L Maximum occurs when L -b2A -602-1 30----Maximum Area occurs when Length 30 and Width 30 ---. In this case the maximum area is 400 ft2 Since this is not in calculus Ill provide a non-calculus answer. Expected_optimum p print Brute Force t.

About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy Safety How YouTube works Test new features Press Copyright Contact us Creators. Record the length width area and perimeter of each rectangle. Perimeter of rectangle 2 l b Where l is the length.

For any rectangle the area is calculated by multiplying the length by the width eg. Or dAdx 200 - 2x. Perimeter P means adding up all 4 sides of a rectangle or PwwLL2wL where w is the width and L is the Length.

Compare your data to your prediction in 1. Therefore the maximum area of the rectangle is 25 square units. The value of the area A at x 100 is equal to 10000 mm 2 and it is the largest maximum.

B is the breadth. In order to have a perimeter of 100 feet that means that. A circle gives the maximum area for a given perimeter.

Therefore the dimensions of the rectangle are l 5 units and b 5 units. Fixed perimeter coordinate graphs will be completed recording the bottom edge and area ordered pairs separate graphs for P 12 and P 24. Hence the maximum area of a rectangle with given perimeter is equal to ceil perimeter4 floor perimeter4.

You can divert from this and see if the area gets any larger or you can use the mathematical way. Area A of the rectangle x200-x. Bottom edge side edge perimeter and area are recorded for all possible perimeter of 12 and perimeter of 24 rectangles.

Length i breadth half-length a area length breadth if a max_area. Since every rectangle has four sides if you know the perimeter divide it by four to find the length of each side. Then its breadth perimeter2 - length.

Or 0 2002x. Find the maximum area of a rectangle with a perimeter of 54 centimeters. A rectangle will have the maximum possible area for a given perimeter when all the sides are the same length.

Substituting the value of b 5 in equation i we get l 5 10 l 5. For maximum or minimum putting dAdx 0. The maximum area for a rectangle of fixed perimeter is that of the square that can be formed with the given perimeter.

For area to be maximum of any rectangle the difference of length and breadth must be minimal. Then find the area by multiplying the length times the width. The points can be connected to form.

The perimeter is found by adding all of the sides together ie. We know the rectangle always has a perimeter of 80 so 2l2w80 which simplifies to be lw40. A rectangle of 10 metres by 20 metres would have an area of 10 x 20 200 m 2.

How much fence is. 1 - Solve the same problem as above but with the perimeter equal to 500 mm. If the length x and the width y then the perimeter P40 P2x2y40-xy20-y20-x As for the area A.

The pentagon that gives the maximum area for a given perimeter is a regular pentagon. A L60-L A 60L - L2-----Rearrange. Substituting the values in equation iii we get A 5 5 25.

The quadrilateral that gives the maximum area for a given perimeter is a square. Below is the implementation of the above approach. Use slider b to create the different rectangles with the perimeter of 24 units.

Maximum Area AwL is when the rectangle is a square or where swL. How do I find the maximum area for a rectangle with a fixed perimeter of 120----Area LW Perimeter 2L 2W-----2L2W 120 L W 60 W 60-L-----Substitute into the Area formula. The shape of these graphs and the information they give will be discussed.

Come up with a. 3 Use the sliders to explore several different perimeters and areas. Solve Study Textbooks Guides.

Maximum Area with Fixed Perimeter If the garden is rectangular it will have the largest possible area when the length equals the width. So if you select a rectangle of width x 100 mm and length y 200 - x 200 - 100 100 mm it is a square you obtain a rectangle with maximum area equal to 10000 mm 2. So in such case the length must be ceil perimeter 4 and breadth will be be floor perimeter 4.

Manish fixed 4 8 poles. Or A 200x -x2. This follows since given a positive number A with.

Let the length of the rectangle is x mm. Half perimeter 2 step_size 01 max_area 0 for i in np. Click hereto get an answer to your question Find the maximum area of the rectangle that can be formed with fixed perimetre 20 units.

Max_area a config length breadth return max_area config p 36 print Expected t.

Maximum Area Of Rectangle Given Perimeter Youtube

Maximizing Area Of 4 Sided Rectangular Enclosures With A Fixed Perimeter Optimization Youtube

How To Optimize The Areas Perimeters Of Rectangles Video Lesson Transcript Study Com

Show That All The Rectangles With A Given Perimeter The Square Has The Largest Area Youtube